How do you add fractions when the denominators are different and one, none or both fractions contain a mixed number?
To add fractions when the denominators are different simply find the lowest common multiple shared by both denominators, increase the fractions to match their new denominators, then add the numerators up, if they exceed the denominator reduce the numerator by the value of the denominator until it
What Is The Denominator?

The denominator is the number seen at the bottom of a fraction. Compared to the number on the top which is labelled as the numerator.
When adding fractions it’s important to ensure that the denominator of both fractions is the same before performing any addition of the two fractions.
You can modify a fraction’s proportion by equally multiplying the numerator and the denominator by the same number. For example, these fractions below are all the same but are written with different denominators:
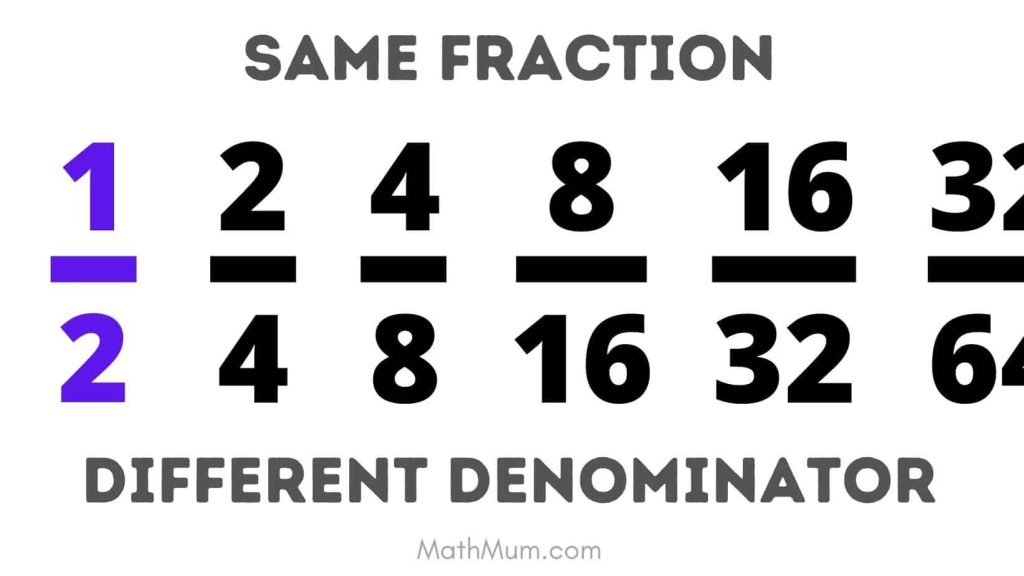
When both fractions have the same denominator then you can perform the next step of adding the fractions together.
Adding Fractions Without Mixed Number
Once the fractions have the same denominator the process of adding now falls on the numerator numbers. Simply add these two numbers together and write that sum as the new numerator value and carry over the current denominator value. This is your solution.
This is how the process works with a common fraction question below:
$$ \frac{3}{4} + \frac{5}{6} $$
As you can see from the above question the two denominators are different. The first question you need to ask is what is the lowest common multiple of both numbers?
Here are the multiples of 4 and 6:
$$ 4 \space {4, 6, 8, \fcolorbox{blue}{aqua}{12}, 16, 20, \fcolorbox{blue}{aqua}{24},…}\6\space{6, \fcolorbox{blue}{aqua}{12}, 18, \fcolorbox{blue}{aqua}{24}, …} $$
As you can see the commonly shared multiples of 4 and 6 are $12$ and $24$, but what is the lowest or smallest multiple of those two numbers? $12$.
To make the denominators the same you need to change the proportion of the whole fraction so that they both have 12 as their denominator.
To modify the fraction with $4$ you would need to multiply its numerator and denominator by $3$, like so:
$$ \frac{3}{4} \times \frac{3}{3} = \frac{3 \times 3}{4 \times 3} = \frac{9}{12} $$
When multiplying fractions simply multiply the two numerators together to form the new numerator and do the same with the two denominators, multiply them together to form the new denominator.
Apply the same process for the other fraction with a denominator of $6$ – what must you multiply $6$ by to get it to $12$? You would need to multiply it by $2$.
Therefore, the second fraction would change to the following:
$$ \frac{5}{6} \times \frac{2}{2} = \frac{5 \times 2}{6 \times 2} = \frac{10}{12} $$
Once you have your two new fractions your original question will have changed to the following, and applying the addition process to the numerators would result in:
$$ \frac{9}{12} + \frac{10}{12} = \frac{19}{12} $$
As you can see the numerators are added together which form the new numerator and the new denominator (which is now common to both fractions) is moved across without change.
Change Fraction To Mixed Fraction
If the numerator is larger than the denominator then you may need to change your solution to a mixed fraction.
What is a mixed fraction?
A mixed fraction is a fraction that contains a numerator, a denominator and a number outside of the fraction and located to the left of the fraction. It looks something like this:
$$ Mixed\space Number\space \frac{numerator}{denominator} $$
To change a fraction to a mixed number the numerator needs to be larger than the denominator, otherwise there can be no mixed fraction.
Provided the numerator is greater than the denominator the mixed number is the multiple of times the denominator goes into the numerator.
Returning to the solution above, the number of times the denominator $12$ goes into the numerator $19$ is once. Therefore, the mixed number becomes $1$. As there is a remainder of $7$ from the division of $12$ into $13$ this number becomes the new numerator.
Here’s the solution from above now in mixed fraction format:
$$ \frac{19 \div 12 = 1\space r7}{12} = 1\space \frac{7}{12} $$
How To Add Fractions With Mixed Fractions?
To add fractions that contain mixed fractions the same process is used as demonstrated above: first add the fraction portions together ensuring the denominators are the same.
$$ \frac{7}{9}\space +\space 2\space\frac{5}{6} $$
In the example above the denominators are $9$ and $6$. The lowest common multiple of these two numbers is $18$, therefore the fraction portions change to the following:
$$ \frac{14}{18} \space + \space 2\space \frac{15}{18} $$
Notice the mixed number did not change. The fractions are changed so that the addition process can happen.
Now, just focussing on the fraction portion of the question, adding those fractions together produces:
$$ \frac{14}{18} + \frac{15}{18} = \frac{29 \div 18 = 1\space r11}{18} = 1\space \frac{11}{18} $$
The result from adding the fractions first produces a mixed fraction itself. From the mixed fraction result the last step needed is to add the mixed numbers in the original fractions. In this example there is only the number 2, therefore, the final result would be as follows:
$$ 2 \space + \space 1\space \frac{11}{18} = 3\space \frac{11}{18} $$
As you can see from the above, the mixed numbers when added together form a new mixed number and the fraction portion remains the same.
How To Add Fractions With Two Mixed Numbers?
Regardless of whether one or both fractions contain a mixed number the process is still the same when adding fractions together: first, focus on the addition of the fraction portion, then add all the mixed numbers together.
Here’s another example where the addition is between two mixed fractions:
$$ 3\space \frac{2}{5} \space + \space 6\space \frac{5}{7} $$
Step One: Extract the fraction portions from both numbers and add them together:
$$ \frac{2}{5}\ + \ \frac{5}{7} \ = \ \frac{14}{35} \ + \ \frac{25}{35} \ = \ \frac{39 \div 35 = 1\ r4}{35} \ = \ 1\ \frac{4}{35} $$
Step Two: Extract the mixed number portions and add these together along with any mixed from STEP ONE:
The two mixed numbers in the question are 3 and 6, and with the calculation done in step 1 above, there’s an additional mixed number of 1. Therefore, combining all this into one operation looks like this:
$$ 3 \ + \ 6 \ + \ 1 \ = \ 10 $$
Step Three: Combine the numbers together
Combine the whole number from step 2 with the fraction part of step 1 above:
$$ 10 \ + \ \frac{4}{35} \ = \ 10\ \frac{4}{35} $$
Summary
To add fractions together that have different denominators and have one, none or two mixed numbers work on adding the fraction portions together first (get the denominators the same) then once you have finished adding these two together add all the mixed numbers together.